Upon telling people what she does for a living, Ellen Eischen says she often gets the response, “You must be good at balancing your checkbook.” In reality the University of Oregon mathematics professor specializes in number theory, particularly L-functions and automorphic forms. Number theory, she explains, has its origins in ancient Greece but did not have practical application until computers became popular in the 1970s.
Creativity Counts: Possibilities Shaped by Constraints of Arithmetic is an art exhibit born out of Eischen’s frustration with the stereotype about mathematicians being uncreative types. To counter the perception that math is not creative, Eischen devised a course for undergraduates called Math and the Creative Process. In it, students at all levels of math worked together to ultimately produce art inspired by mathematical processes, especially related to number theory. That art is now on exhibit in the Artist’s Projects Space at the Jordan Schnitzer Museum of Art on the UO campus until July 11.
Eischen consulted with JSMA curator Cheryl Hartup for the show and with improvisation teacher and science communicator Heather Barnes to introduce spontaneity into her classroom. Though most of the pieces were done by math students, she had some extra room and invited a couple of colleagues as well.
Martin H. Weissman, author of An Illustrated Theory of Numbers and visiting professor, has a piece in the show called “36 Epicycles” (digital print on acrylic). His artwork is composed of variously colored squares or “tiles” that elicit a pleasing optical illusion that keeps you looking. Use the QR code to read his description for the math that inspired the image.
“Remainders,” he says, “are the cold left over of grade-school arithmetic. But they are the tastiest morsel for mathematicians.”
He goes on to tell us that remainders can say a lot about prime numbers. And that without prime numbers he could read all our private email messages.
The discussion and illustration of practicality versus creativity is not dogmatic or argumentative, but rather fluid and thought provoking. Each artwork in the show has a story behind it that relates to a mathematical problem or process, history or ideology, and all the descriptions can be accessed with QR code in person in the exhibit or at the virtual gallery tour online.
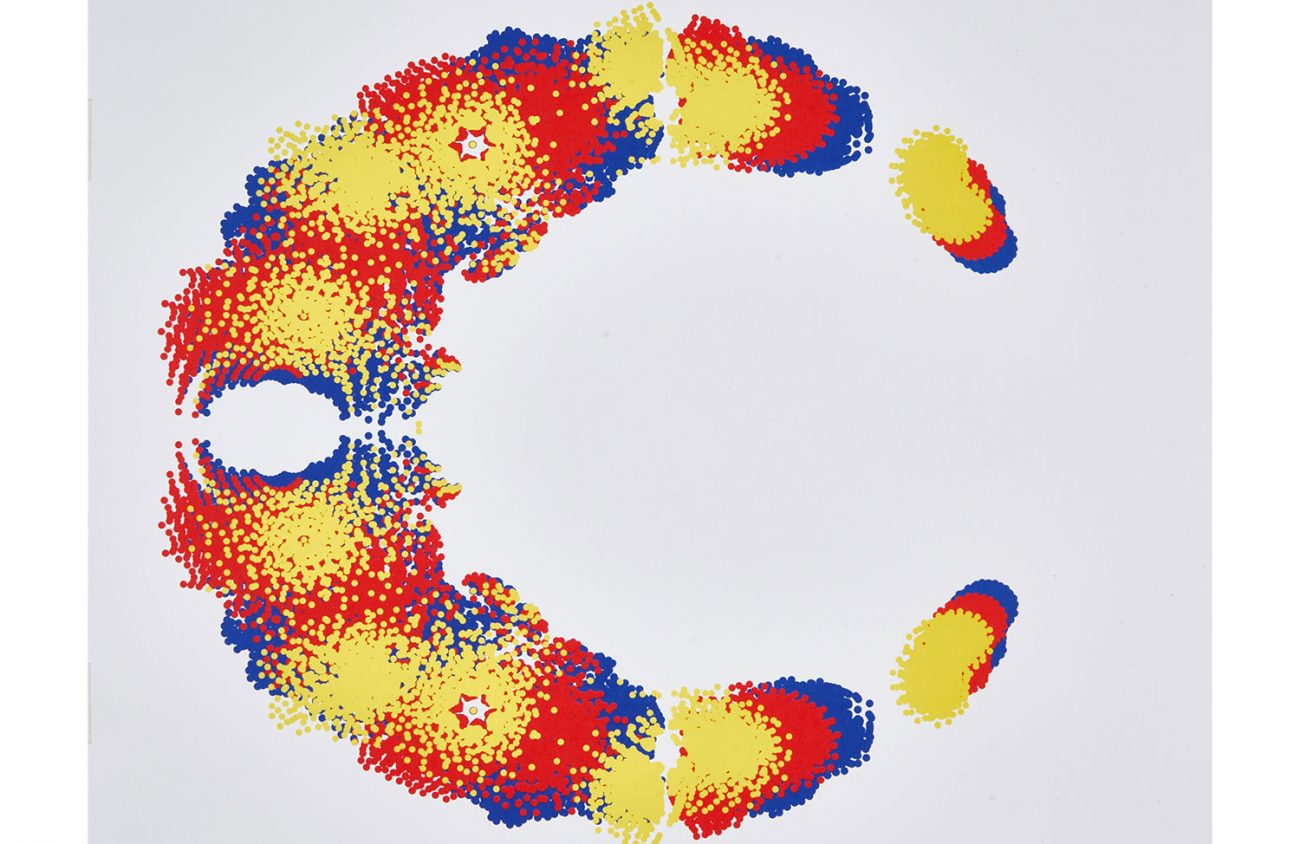
Cruz Godar is a first-year graduate student in the math department. His “Minotaur’s Paradise” is a brilliantly colored digital print on metal. It’s an abstract image but upon closer look, and after reading the description, you see that the entire piece is designed as a maze.
Godar says if you want to create a maze for yourself, use Wilson’s Algorithm.
“It’s a method that makes maze-making as easy as following a recipe, and what’s more, it produces a truly random one — every single possible maze of a fixed size has the same chance of being drawn.”
Undergraduate students Nitan Avivi, Gabby Bennett, Chloe Miller and Azusena Rosales Suares collaborated on a four-piece series of digital prints on paper titled “Constellations of Mathematics.” These light and starry artworks could easily be compared to the ethereal drawing style used by Antoine de Saint-Exupéry for his book The Little Prince, except that they are inspired by roots of polynomials.
Teaching creativity and math on Zoom was not exactly what Eischen had in mind when she first conceived of her course. But the pandemic hit and classes were shut down. Being trained in improvisation, she went with it.
Eischen first became interested in improvisation while doing a postdoctoral fellowship at Northwestern University. She was looking for something to do outside of mathematics and academia, for a change, and took a class at Chicago’s famed Second City. She wound up being involved with improv the whole time she was at Northwestern.
Eischen says that saying “yes and…” is an improvisation technique, but it is also important to be open when working with numbers. Mathematicians often think in terms of what isn’t working. They say no until something works. Improvisation exercises, like finishing each other’s sentences — an exercise Eischen did with her students on Zoom — teaches people to work collaboratively and be in the present.
In her own work in mathematics, Eischen is interested in how L-functions and automorphic forms “link seemingly disparate data.” She wants to find a bridge between the two. Finding commonalities between seemingly different things seems to drive her interest. Whether between art and science or between functions in number theory, she’s always looking for a bridge.
For the virtual tour: JSMA.uoregon.edu/CreativityCounts. The JSMA is now open on Saturday and Sunday from 11-5. Masks are required.